Life-cycle model
We modeled a steelhead population consisting of a total of six age-classes, with four adult ages (3–6). We modeled females only and assumed a 1:1 sex ratio (
Withler 1966;
Ward and Slaney 1988;
Seamons et al. 2004). The model includes repeat spawning at ages four, five, and six because repeat spawning is believed to have been an important characteristic of steelhead life history prior to the major reductions in winter-run steelhead population sizes during the past 40 years (
Hard et al. 2015). The model consists of 19 stages. This results in a square population projection matrix, 19 rows by 19 columns, with fecundity values in the first row and transition rates or zero entries in all other rows. Life stages, transition rates, and fecundities used in the projection matrix and their abbreviations are listed in
Supplementary Material 1, Tables S1 and S2.
Figure 2 shows a schematic of the life-cycle model starting with parr (age 1) and terminating at each adult age (3–6) and life stage or fate (maiden or repeat spawner, surviving to spawn or harvested).
Density dependence is incorporated during the period of freshwater residence prior to smolting in the transition from emergent fry-to-age-1 (parr). Accordingly, the model includes two sub-age-1 life stages: eggs and fry. Fry-to-parr survival (fp) is modeled as a type II (Beverton–Holt) function with fixed parameters
α and
β:
where
α is the inherent maximum fry-to-parr survival rate at low density under optimal conditions,
β is the inverse of the number of fry at which fry-to-adult survival =
α/2, and
n fry is the number of fry. The number of fry is:
where
n eggs is the total egg deposition, sr is the sex ratio, ef is the deterministic egg-to-fry survival rate. Consequently, fp will vary nonlinearly with the number of fry produced by each year’s total spawner abundance as will the number of parr produced the following year by each age and type spawner. All other life-stage transitions are considered deterministic and density independent. The model tracks all life stages from egg deposition to adult return by age and stage class, so that production by each spawner type (age and repeat-spawning status) could be accounted separately.
For modeling convenience, we assume that repeat spawners attempt to repeat spawn the year after their maiden spawning and that there are no third-time spawners. Repeat spawning the year after maiden spawning was found to be the most common pattern in steelhead in Kamchatka as determined by scale analysis of several hundred samples collected by a joint US-Russian conservation research program between 1996 and 2005 (
Pavlov et al. 2001; N. Gayeski, personal observation, 2015). Repeat spawning was modeled for each maiden spawner in age-classes three to five by simply assigning an age-specific probability of surviving spawning to re-enter the ocean. We assumed that there was no difference in reproductive effort between maiden spawners in a given age-class that succeeded in repeat spawning and those that did not. The maximum adult age was set at six and the maturation rate of six-year old fish was set implicitly to 1. Modeling was conducted in MATLAB 7.10.
Puget Sound steelhead in the late 19th century were harvested exclusively in terminal areas (river mouths and associated estuary or nearshore) and in-river fisheries (
Wilcox 1898). Thus, only mature fish, both maiden and potential repeat spawners, were harvested.
Gayeski et al. (2011) argued that given that the peak commercial harvest of steelhead in Puget Sound occurred in 1895, six years after statehood and the initiation of large-scale commercial fishing for steelhead, the total adult return (harvest plus spawning escapement) was likely recruited from a population at or very near its unfished equilibrium abundance, as determined by the average conditions in freshwater and the marine environment that existed in the final quarter of the century.
Gayeski et al. (2011) employed a Bayesian approach to estimate the posterior distribution of the 1895 adult return to the Stillaguamish from the reported commercial steelhead catch for the Stillaguamish augmented by estimates of unreported, non-commercial in-river harvest of steelhead by settlers and others based on historical records and reports. The commercial harvest data were reported in pounds, which Gayeski et al. converted to estimates of total numbers landed using a distribution of average steelhead weights estimated for the period. The total return was then estimated from the estimated total numbers landed and an estimate of the distribution of the total harvest rate assuming a binomial distribution for the harvest process. The posterior mode (single most probable value) of the estimated total adult return in 1895 was 69 200 corresponding to an estimate of 34 600 adult females assuming a sex ratio of 1:1.
Gayeski et al. (2011) estimated that this return was most likely harvested at a rate of nearly 55%, yielding a total harvest of females of nearly 18 900 and a spawning escapement of approximately 15 700. We assumed that this was the case and employed the life-cycle model to estimate the number of age-1 individuals that must have been produced in order to recruit a female population of 34 600. We did this by running the model for 25 time steps (years) starting with 10 years of no harvest and adding harvest at time step 11 that built up steadily to a maximum rate of 0.545 at time step 16 equal to the harvest rate on the 1895 population estimated by
Gayeski et al. (2011). The model was run for an additional 9 time steps to verify that the year 16 total catch was a maximum, reflecting the historical harvest record (
Fig. 3). The density-dependence capacity parameter was adjusted to achieve the estimated 1895 total catch and total spawner abundance, as described below. The model was then run with the harvest rate set to zero to determine the total unfished equilibrium abundance of all ages at the stable age distribution. We refer to this henceforth as the 1895 equilibrium.
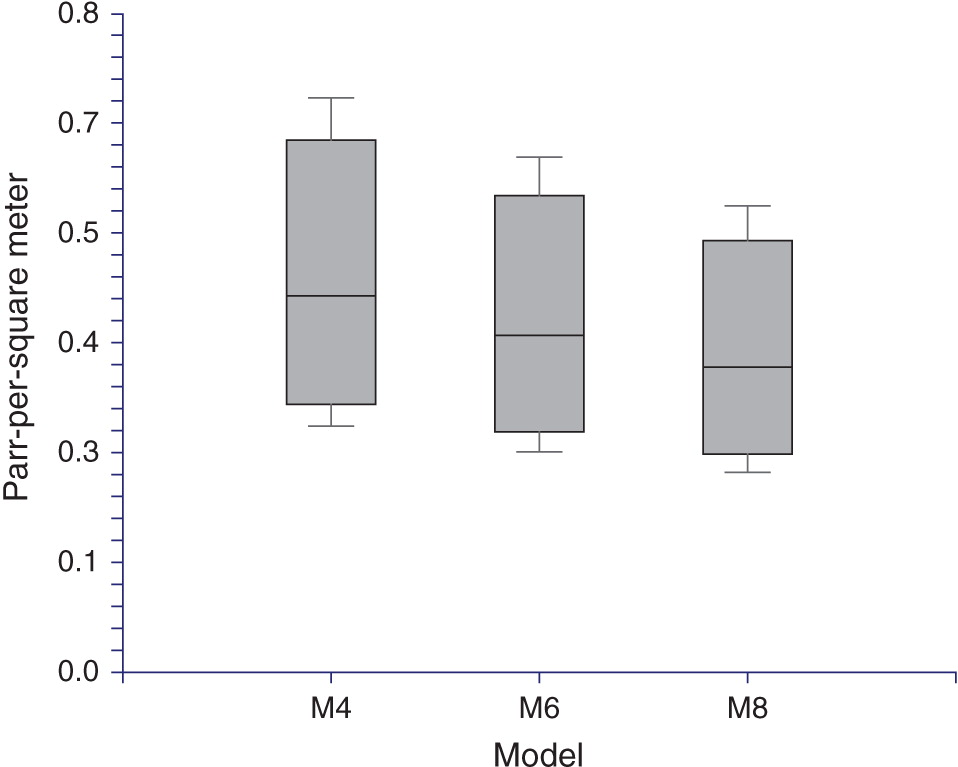
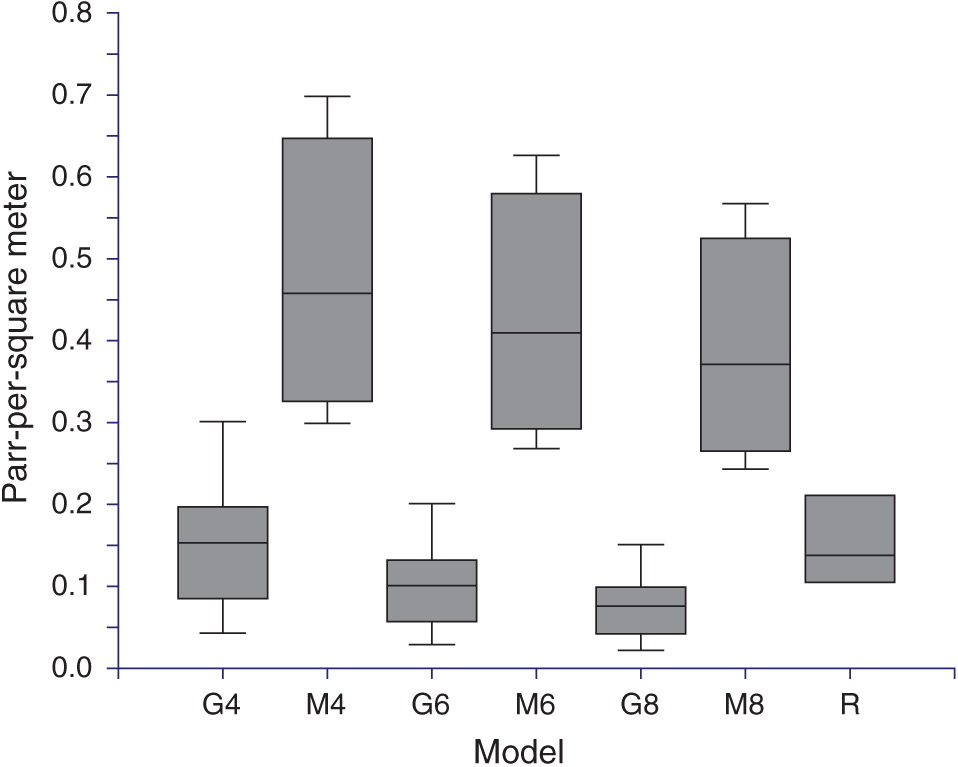
We tracked the annual abundance of harvested and unharvested maiden and repeat spawners separately in order to evaluate the impact of harvest on age-classes and on maiden and repeat spawners. The model has 19 stages, including 17 stages for the four oldest age-classes, of which 14 stages are matures. These enable the model to keep track of all spawner life histories and the life histories of all harvested matures (
Fig. 2,
Table S2).
Fertilities, also referred to as “effective fecundities,” are measured as the number of age-1 progeny (parr) produced by each spawner of a particular age and type,
x; that is, the number of offspring surviving to year
t + 1 produced by each age-
x spawner in year
t. These numbers occupy the first row of the square population projection matrix. Thus, the model assumes a prebreeding census, whereby parents are counted each year prior to spawning and their offspring are counted prior to spawning the following year. Fertilities are, therefore, matrix elements generated by underlying vital rates. The vital rates are fecundity (eggs/age and type female), sex ratio, egg-to-emergent fry survival, and density-dependent fry-to-parr survival (
Supplementary Material 1, Table S2). Fecundity was treated as age- and size- (weight)-specific for both maiden and repeat spawners in each mature age-class. Since repeat spawners must recover body condition upon re-entering marine waters before they invest energy in gonad production and because they have less time to feed in marine waters before returning to freshwater to spawn than maiden fish of the same age, we assigned repeat spawners a fecundity value slightly greater than maiden spawners in the previous age-class (to account for some body size increase) but less than the value assigned to maiden spawners in the same age-class.
Age-specific weights and fecundities are based on length, weight, and fecundity data collected from steelhead in western Kamchatka on the Russian North Pacific Rim in 2001–2004 (N. Gayeski, personal observation, 2015), and scale analyses of the same individuals made by Dr. Kiril Kuzhichin at the Department of Ichthyology, Moscow (Russia) State University. We used data from Kamchatka because we had fecundity (egg number) and length, weight, and age data for each of approximately 80 individual females. We selected representative lengths-at-age for 3-, 4-, 5-, and 6-year-old maiden and repeat-spawning females that provided representative weights for given lengths and ages. We compared both measured and estimated fecundity-at-length from the Kamchatka data to length-fecundity data for several British Columbia steelhead populations provided to the lead author by Dr. Bruce Ward to evaluate how representative of North American steelhead Kamchatka steelhead are. Kamchatka steelhead had slightly greater fecundity at a given length and weight than the majority of British Columbia winter-run populations. We then adjusted the expected fecundity-at-length downward by adjusting the intercept of the natural log–log regression of fecundity on length of the Kamchatka samples to obtain an average fecundity approximately equal to the mean value reported by
Quinn (2005, Table 15-1) that was based on a survey of available published and unpublished data, including the British Columbia data that we examined. We expected that the average fecundity value reported in
Quinn (2005) was more likely than the unadjusted Kamchatka data to be representative of Puget Sound steelhead in the late 19th century, as indicated by the range of average steelhead weights reported by
Gayeski et al. (2011). The resulting parameterization of the model was corroborated by the model’s achieving the target average adult weight of Puget Sound winter-run steelhead of 8.25 lbs. estimated by
Gayeski et al. (2011). Length-weight and length-fecundity equations are provided in
Supplementary Material 2. Age-specific fecundities are listed in
Supplementary Material 1, Table S2.